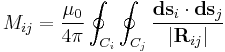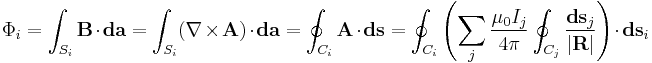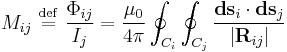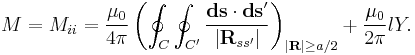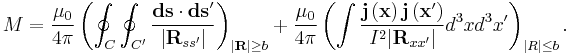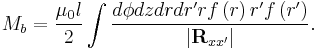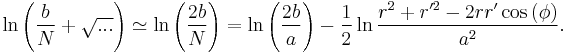Derivation of self inductance
The mutual inductance by circuit i on circuit j is given by the double integral Neumann formula
Contents |
Derivation
where
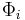 is the magnetic flux through the ith surface by the electrical circuit outlined by Cj
is the magnetic flux through the ith surface by the electrical circuit outlined by Cj- Ci is the enclosing curve of Si.
- B is the magnetic field vector.
- A is the vector potential. [1]
Stokes' theorem has been used.
so that the mutual inductance is a purely geometrical quantity independent of the current in the circuits.
Self inductance
In the self inductance case 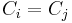 . Therefore 1/R diverges and the finite radius of the wire and the distribution of the current in the wire must be taken into account. A generic formula for the self inductance
. Therefore 1/R diverges and the finite radius of the wire and the distribution of the current in the wire must be taken into account. A generic formula for the self inductance  is available provided that the length
is available provided that the length  of the wire is much larger than its radius
of the wire is much larger than its radius  :
:
Points with 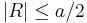 now must be excluded in the curve integral. The correction term proportional to
now must be excluded in the curve integral. The correction term proportional to  originates from short wire segments which are essentially cylinders.
originates from short wire segments which are essentially cylinders. 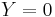 if the current flows in the surface of the wire,
if the current flows in the surface of the wire, 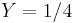 if the current is homogeneous in the wire.
if the current is homogeneous in the wire.
For a derivation start from a slight generalization of the curve integral for mutual inductance,
Here j(x) is the current density, I is the total current, and b is a length according to  . The idea is to calculate the contribution from points with |R|> b with the curve integral, and to use the actual current density for the contribution
. The idea is to calculate the contribution from points with |R|> b with the curve integral, and to use the actual current density for the contribution  from points with |R|
from points with |R|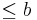 . Let the current density in a wire segment be given by
. Let the current density in a wire segment be given by  , where r is a radial coordinate, and
, where r is a radial coordinate, and  is a unit vector along the axis. Then, using cylinder coordinates and performing the trivial integrations over
is a unit vector along the axis. Then, using cylinder coordinates and performing the trivial integrations over 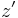 and
and 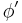 ,
,
The integral over z (from -b to b) may be performed using 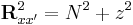 ,
, 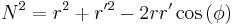 ,
,
Because of  the logarithm may be replaced by
the logarithm may be replaced by
In the realistic cases 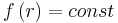 and
and 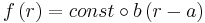 the remaining integrals now either are trivial or may be looked up in an integral table. The result is
the remaining integrals now either are trivial or may be looked up in an integral table. The result is 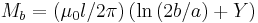 . Finally, the self inductance cannot depend on the arbitrary length b, which is the lower integration limit for the curve integral and the upper integration limit for
. Finally, the self inductance cannot depend on the arbitrary length b, which is the lower integration limit for the curve integral and the upper integration limit for  . Therefore the contribution proportional to
. Therefore the contribution proportional to 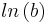 cancels against a contribution from the curve integral, so that one now also may choose
cancels against a contribution from the curve integral, so that one now also may choose  to simplify the result.
to simplify the result.
Error estimation and restrictions
Wire segments of length b aren't perfect cylinders, they typically are curved by an angle 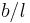 . The scalar product between current elements thus contains an additional factor of order
. The scalar product between current elements thus contains an additional factor of order 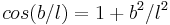 . Endings of wire segments aren't perfectly parallel. The maximal length of such an ending is a, leading to a relative error a/b. A typical tilt angle only is b/l, leading to a factor
. Endings of wire segments aren't perfectly parallel. The maximal length of such an ending is a, leading to a relative error a/b. A typical tilt angle only is b/l, leading to a factor 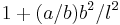 . The curve integral uses distance along the axis instead of the distance between points anwhere in the wire. This contributes at most a factor
. The curve integral uses distance along the axis instead of the distance between points anwhere in the wire. This contributes at most a factor  . In total, the expression is wrong by a factor
. In total, the expression is wrong by a factor  , which is small for
, which is small for  .
.
In the skin effect case another approximation is hidden in the assumption of constant current density. This may lead to wrong results because additional currents flow in the surface of wires close to each other (expelling the magnetic field). In this case the Laplace equation must be solved to determine currents and fields.
References
- ^ Jackson, J. D. (1975). Classical Electrodynamics. Wiley. pp. 176, 263.